3. The Lyapunov exponent.

We define f_n(x) for a given function f(x) and a given starting value x₀ by

 

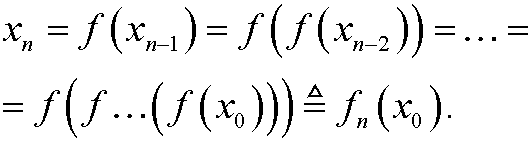
By the chain rule we then have that

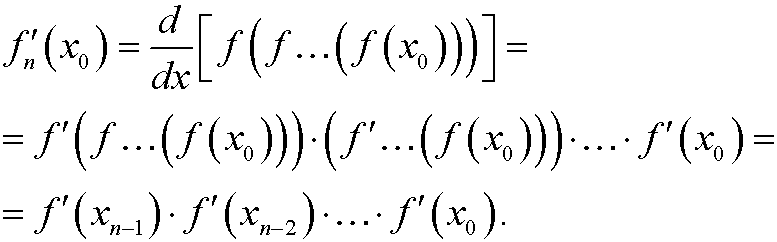
By taking logarithms and dividing by n on both sides we get

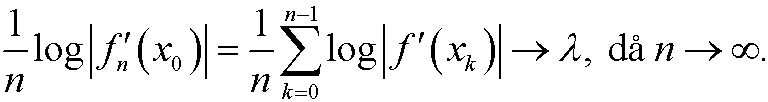
This convergence can be proved by using a particular mathematical technique. The limit  is called the Lyapunov exponent of the dynamical system.

Now suppose that we have two (close) starting values x₀ and y₀. By using the mean value theorem we see that

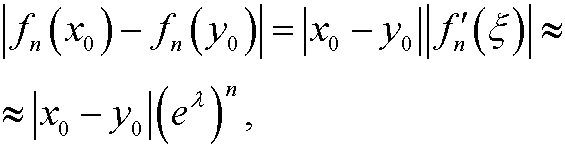
since

 

and

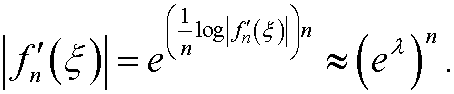
The conclusion is that 

a) if  we have that 

 

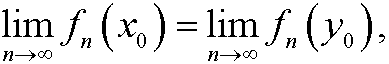
that is, the system is not sensitive to the starting value.

b) if  then

 

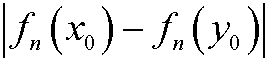
diverges to infinity with exponential growth.